Inter-specific Competition

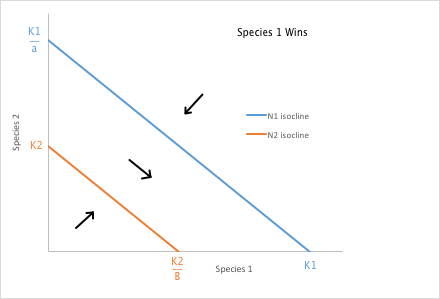
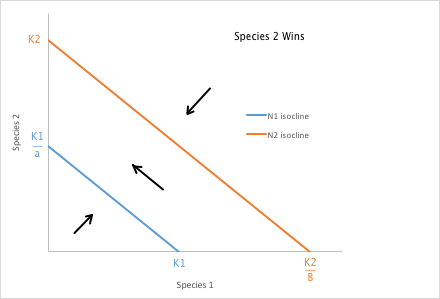
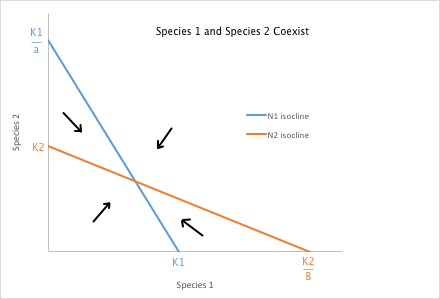
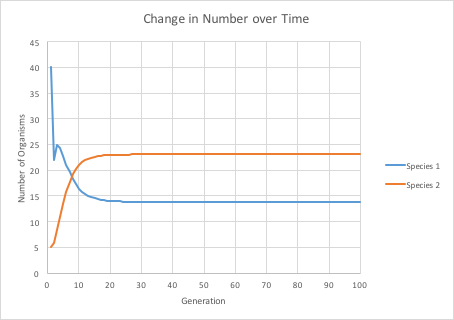
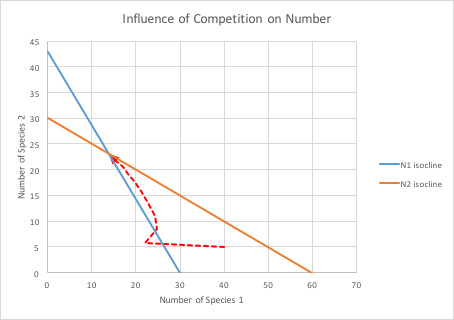
As we learned from the bird beak lab, inter-species competition for a finite resource can be quite intense, and can result in partitioning of a niche or local extinction of the less successful species. We can model these interactions mathematically. Remember the logistic growth equation? That's the starting point for these calculations. If there is no competition between two species, then they should grow in number until they reach the carrying capacity of the local environment. However, if a second species is interfering with the first, then the first species will be less successful and, depending on the degree of interference or inhibition, the first species might die out or stabilize at a lower number. It is also possible that the first species interferes with the second, so both species may be negatively affected by the competition with each other for a limited resource. Here, then, are the equations for Species 1 and Species 2. The new terms, αN2 and βN1 serve to reduce the number of the opposite species based on how badly the competing species inhibits the other (the α and β terms) multiplied by the number of individuals of the competing species. Note that if either the competition factor is zero or the number of competitors present is zero, this term drops out and the other species reaches its carrying capacity.

Species 1: ΔN1 = r1 N1 ((K1 - N1 - αN2 ) / K1) where α = negative effect of Species 2 on Species 1

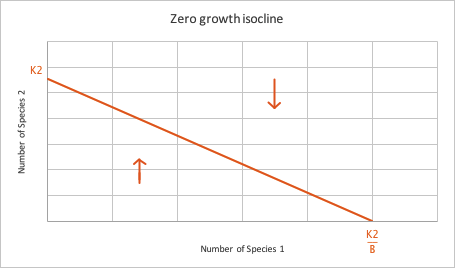
Species 2: ΔN2 = r2 N2 ((K2 - N2 - βN1 ) / K2) where β = negative effect of Species 1 on Species 2

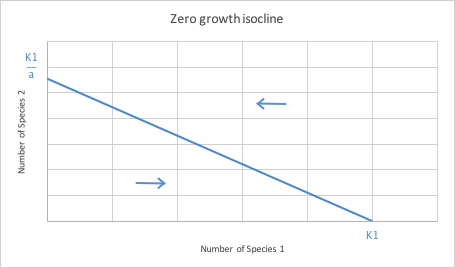
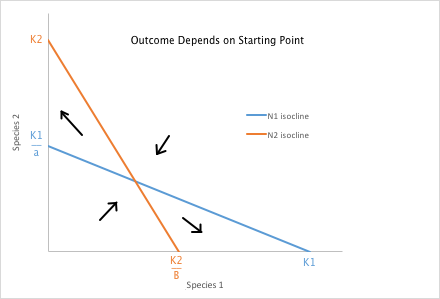
Species 1 Isocline: How can we figure out when a species will increase in number and when it will decrease? If Species 2 is not part of the picture (N2=0) there is no suppression due to competition, so the growth or decline of Species 1 is determined only by how many there are currently, and by the carrying capacity of Species 1. If there are more of Species 1 than the carrying capacity (N1>K1) then species 1 will decline. If there are fewer of species 1 than the carrying capacity (N1<K1) then species 1 will increase. If species 1 is at the carrying capacity (N1=K1) then there will be no change in number. On a graph of Species 1 vs Species 2, the carrying capacity of Species 1, K1 is the x-intercept of a line. But at what number of Species 2 is there zero growth of Species 1? We can solve for the y-intercept of our zero-growth line by plugging in a zero for N1 (where the number of Species 1 is not changing) and solving for N2

(K1 - N1 - αN2) = 0

K1- αN2 = 0

K1= αN2

K1/α = N2

Conclusion: For any x-y value of N1 and N2 that is above the Species 1 zero growth isocline, N1 will decrease. For any value of N1 and N2 that is below the zero growth isocline, N1 will increase.

Species 2 Isocline: As we did above for Species 1, we can also determine when the number of species 2 will increase and decrease. Because the number of Species 2 is graphed on the y-axis, the graph is flipped. The carrying capacity of Species 2, K2, is the y-intercept. We calculate the x-intercept, the point at which the number of species 1 causes zero growth of species 2, below, by setting N2 = 0:

(K2 - N2 - βN1) = 0

K2 - βN1 = 0

K2 = βN1

K2/β = N1

Conclusion: For any x-y value of N1 and N2 that is above the Species 2 zero growth isocline, N2 will decrease. For any value of N1 and N2 that is below the zero growth isocline, N2 will increase.

When we overlay the two zero-growth isoclines on the same graph, there are four possible outcomes.

  1. When the Species 1 isocline is above the species 2 isocline, Species 1 eventually drives Species 2 extinct and Species 1 reaches its carrying capacity.
  2. When the Species 2 isocline is above the species 1 isocline, Species 2 eventually drives Species 1 extinct and Species 2 reaches its carrying capacity.
  3. In the third scenario, the isoclines cross, and one species will go extinct. The outcome is an unstable equilibrium and depends on the initial numbers of Species 1 and 2.
  4. In the fourth scenario, the outcome is a stable equilibrium, and the end result is coexistence, regardless of the initial numbers of Species 1 and Species 2.

For a more detailed explanation of these graphs, please watch this video.

To explore these graphs with different values of N1, N2, K1, K2, α and β, download the Competition Excel simulation. excel iconBelow is an example. The red line in the second graph indicates the change in number of Species 1 plotted against Species 2 and, in this case, leads to a stable equilibrium.

  N1 K1 α N2 K2 β
  Number of Species 1 Carrying Capacity Species 1 alpha (effect of 2 on 1) Number of Species 2 Carrying Capacity Species 2 beta (effect of 1 on 2)
Initial 40 30 0.7 5 30 0.5
Final 13.8 30 0.7 23.1 30 0.5