SET THEORY Part 1
Terms and Problems, Diatonic Sets
1. Terms:
| setsubset | diatonic set | interval set | |
| interval inventory (interval vector) | |||
| permutations and combinations | |||
| trichord | chromatic set | ||
| pentachord | |||
| tetrachord |
2. How many ways can the notes of a particular trichord be combined? a tetrachord? a pentachord? What are the arithmetical expressions of the possible combinations of notes in three, four, five, and six-note patterns?
3. Self-help problems. Solutions follow.
Problem 1: Write all the permutations of this tetrachord (Hint: rotate the pattern three times. Next, switch a pair of notes. Write the resulting pattern at each *. Rotate each new combination three times.). Test the following statement with a spot check interval inventory: all 24 patterns belong to the same class of tetrachord because all have the same interval content.
| 1* | E | F | G | A | 9* | 17* | ||||||||||
| 2 | 10 | 18 | ||||||||||||||
| 3 | 11 | 19 | ||||||||||||||
| 4 | 12 | 20 | ||||||||||||||
| 5* | 13* | 21* | ||||||||||||||
| 6 | 14 | 22 | ||||||||||||||
| 7 | 15 | 23 | ||||||||||||||
| 8 | 16 | 24 |
Problem 2: Complete an interval inventory (interval vector) of this arrangement of white keys to see if the interval content changes or remains the same as other versions of the set of white keys. Use example 5 as a model.
| F | G | A | B | C | D | E | m2 /M7 | M2 /m7 | m3 /M6 | M3 /m6 | P4 /P5 | tt |
| G | A | B | C | D | E | |||||||
| A | B | C | D | E | ||||||||
| B | C | D | E | |||||||||
| C | D | E | ||||||||||
| M2 | M3 | 1 | 1 | |||||||||
| D | E | |||||||||||
| total |
Problem 3: Complete an interval vector of this pentachord. Use example 5 as a model.
| D | E | G | A | C | m2 /M7 | M2 /m7 | m3 /M6 | M3 /m6 | P4 /P5 | tt |
| E | ||||||||||
| G | ||||||||||
| A | ||||||||||
| total |
Solution 1: Answers will be in this approximate order if the hint was followed. The arrows indicate which note was shifted create a new permutation of the basic tetrachord. Any solution pattern is acceptable so long as it is efficient and organized.
| 1* | E | F | G | A | 9* | E | G |
F
|
A | 17* |
A
|
F | G | E | ||
| 2 | F | G | A | E | 10 | G | F | A | E | 18 | F | G | E | A | ||
| 3 | G | A | E | F | 11 | F | A | E | G | 19 | G | E | A | F | ||
| 4 | A | E | F | G | 12 | A | E | G | F | 20 | E | A | F | G | ||
| 5* | F |
E
|
G | A | 13* | E | F | A |
G
|
21* | F |
E
|
A |
G
|
||
| 6 | E | G | A | F | 14 | F | A | G | E | 22 | E | A | G | F | ||
| 7 | G | A | F | E | 15 | A | G | E | F | 23 | A | G | F | E | ||
| 8 | A | F | E | G | 16 | G | E | F | A | 24 | G | F | E | A |
Solution 2: The interval content matches that of CDEFGAB. In fact, this inventory remains unchanged regardless of the order in which the white keys are presented. This remains true under every diatonic key signature.
| F | G | A | B | C | D | E | m2 /M7 | M2 /m7 | m3 /M6 | M3 /m6 | P4 /P5 | tt |
| M2 | M3 | tt | P5 | M6 | M7 | 1 | 1 | 1 | 1 | 1 | 1 | |
| G | A | B | C | D | E | |||||||
| M2 | M3 | P4 | P5 | M6 | 1 | 1 | 1 | 2 | ||||
| A | B | C | D | E | ||||||||
| M2 | m3 | P4 | P5 | 1 | 1 | 2 | ||||||
| B | C | D | E | |||||||||
| m2 | m3 | P4 | 1 | 1 | 1 | |||||||
| C | D | E | ||||||||||
| M2 | M3 | 1 | 1 | |||||||||
| D | E | |||||||||||
| M2 | 1 | |||||||||||
| total | 2 | 5 | 4 | 3 | 6 | 1 |
Solution 3:
| D | E | G | A | C | m2 /M7 | M2 /m7 | m3 /M6 | M3 /m6 | P4 /P5 | tt |
| M2 | P4 | P5 | m7 | 2 | 2 | |||||
| E | G | A | C | |||||||
| m3 | P4 | m6 | 1 | 1 | 1 | |||||
| G | A | C | ||||||||
| M2 | P4 | 1 | 1 | |||||||
| A | C | |||||||||
| m3 | 1 | |||||||||
| total | 3 | 2 | 1 | 4 |
Problem 4: Complete an interval vector of this pentachord. Use example 5 as a model.
| A | C | D | E | G | m2 /M7 | M2 /m7 | m3 /M6 | M3 /m6 | P4 /P5 | tt |
| C | ||||||||||
| D | ||||||||||
| E | ||||||||||
| total |
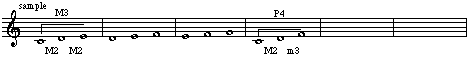
Problem 5: This exercise will produce one example of each type of trichord inherent in a diatonic mode. Each is a one-of-a-kind series of intervals. Each can be transposed at least once without adding sharps or flats. Using only natural pitches (white key notes), write one example of each type of trichord having a range of P5 or less. Do not duplicate an interval sequence (i.e. once M2-m2 has been used, do not use it again). Label the intervals in each trichord. When writing these patterns, consider the melodic potential of each pattern. Each pattern can be rotated, reordered, and transposed to produce a melody based on varied repetitions of a thematic cell.
![]()
![]()
Problem 6:
Write four different versions of the interval sequence P4-M2 using only natural pitches. Measure 1 is the original pattern.
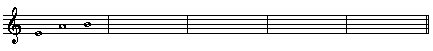
Solution 4: Compare the results of Solution 3 and Solution 4.
| A | C | D | E | G | m2 /M7 | M2 /m7 | m3 /M6 | M3 /m6 | P4 /P5 | tt |
| m3 | P4 | P5 | m7 | 1 | 1 | 2 | ||||
| C | D | E | G | |||||||
| M2 | M3 | P5 | 1 | 1 | 1 | |||||
| D | E | G | ||||||||
| M2 | P4 | 1 | 1 | |||||||
| E | G | |||||||||
| m3 | 1 | |||||||||
| total | 3 | 2 | 1 | 4 |
Solution 5: Sample solutions. Each of these patterns is a one-of-a-kind sequence of intervals. All can be transposed at least one time without adding any sharps or flats. For example, the pattern in measure 4 occurs at two different levels among the white keys. The pattern in measure 7 occurs at four different levels. All patterns with the same combination of intervals are like objects and thus belong to the same set of sets.



Any of these melodic cells can be transposed and reordered to create a cohesive thematic flow in a composition or an improvisation. Experiment with a few of these cells. Improvise a melody in which the original idea is followed by a retrograde transposition, a transposed original by another retrograde transposition, etc. Try a series of other kinds of variants. Later in this chapter, you will be asked to compose a melody based upon trichord cells. This method of composing can be used to create diatonic, chromatic, or fully twelve-tone melodies.
Solution 6: All P4-M2 inherent in the natural pitches.
